
my Theory Of Everything: Fractal æther
David Bohm died on October 27th, 1992.
That day, he was working with his longtime collaborator Basil Hiley at birkbeck College, London.
Just before he left his office,
he telephoned his wife and was quite excited, saying…
“I feel I’m on the edge of something…”
Did Bohm see fractals (and their relevance to his theory) ?
Like in the famous book “Chaos: Making a New Science” book by James Gleick from 1987 ?
Or the do-it-yourself books like: “Fractal Programming in C” by Roger T. Stevens from 1989?
My “fractal aether” theory is connecting Bohm’s ideas with fractals.
Part 1 – David Bohm meets Benoit Mandelbrot
In the wonderful movie: “Infinite Potential: The Life & Ideas of David Bohm” from 2020, we see that the particles are moving inside a “track” made of potential, just like a river flows inside a valley.
Just like if we look down from an airplane on a river (or look on a map of the river) we see the river moving in 2 dimensions, but actually in reality the river’s movement is caused by the 3rd dimension of height difference (mountains and valleys);
So do all the particles in our reality seem to us to be moving in 3 dimensions (that we can see), but actually in reality the particles’ movement is caused potential differences (that we cannot see).
Since we humans can’t imagine the 4th dimension, the following discussion will be as if we move in 2 dimensions and as if our movement is caused by the 3rd dimension.
Please note: in every instance when I say 4th dimension, I mean 4th spatial dimension. So if you consider time as the 4th dimension then you should read what I say as 5th dimension.
So there is a “raised-relief map”
https://en.wikipedia.org/wiki/Raised-relief_map
that is “sitting” under our observed reality and is effecting our movement, and this map is like a “fractal landscape”,
https://en.wikipedia.org/wiki/Fractal_landscape
except that it’s not based on random numbers – instead it’s based on an actual fractal like the Mandelbrot set.
https://en.wikipedia.org/wiki/Mandelbrot_set
But in our discussion here, instead of talking about the height (altitude) or depth at a certain point on the map, I will talk about the color at that point, because we are used to see the Mandelbrot set as a colorful painting and not as a sculpture.
Part 2 – How my theory fits with General Relativity
Ok here instead of telling you what’s good about my theory I will first tell you why my theory is not bad. The reason being that some people might see the word “aether” and freak out and stop reading.
First of all each time we talk of “space curvature” we simply stretch the painting of our underlying map (like our Mandelbrot set looks in a distorting mirror https://en.wikipedia.org/wiki/Distorting_mirror ).
Michelson–Morley experiment
This experiment checks whether there is absolute space, but the experiment depends on the aether staying unchanging.
The prerequisite (precondition) for this experiment to be meaningful is for the aether itself not to know what is the speed (of the particle) now, and so the aether does not adjust itself.
But that’s exactly what happens with the fractal aether, it changes in every moment according to the conditions in that instant (including the speed).
Explanation to the speed of light limit:
When we look at the Mandelbrot set, we see that there are places with lots of details where there is a lot to compute (these places are on the edge of the Mandelbrot set).
Slow particles pass through places where there isn’t many details to calculate, so their speed isn’t slowed down by much.
On the other hand, fast particles pass through places where there’s a lot to calculate (on the edge of the Mandelbrot set where there is the most detail) so their speed is slowed down more and more.
The faster they go the more they are slowed down, so this balances around the “speed of light” which they can’t go over.
Why does the light (fast movement) pass in a place where there are more calculations, and on the other hand a particle with mass (slow movement) passes in easy to compute places?
Because the light itself causes the whirlpools (vortexs), the places with the intense calculations.
Just like when a river is flowing slowly the flow is smooth (laminar) but when the flow is faster and faster there is more and more turbulence like “white water”.
The faster the movement is the more layers of fractal aether that the particle is going through. The friction in them causes the slowdown (more calculations to get to the next pixel).
Extra thought:
How much is the movement slowed down? What eventually dictates the “speed of light”?
It comes from the “refresh rate” of the universe. The “speed of light” is the distance of one “pixel” in the time that it takes for the universe to refresh the whole page.
Mach’s principle (rotating room)
Einstein solved this by deciding that only relative motion exists, and absolute motion does not exist. But I think this is not correct.
The universe DOES communicate with itself and check which part is rotating (communication that is faster than the speed of light).
So this is solved here by the Universe communicating all the time with itself instantaneously.
Properties of the aether (in classical theory)
On the one hand it should be very rarified (thin) not to interrupt the movement of massive objects like the Earth.
On the other hand it must be rigid (stiff) in order to allow for the movement of waves.
Here with the fractal aether these properties are not relevant anymore:
The fractal aether is “programmed” to effect only small particles which are close to the speed of light.
It’s like movement inside a viscous liquid which is “Non-Newtonian fluid” like for example honey or custard – a slow movement is possible but a fast movement is not.
Low speeds like the Earth’s speed do not create interaction with the aether (or create a negligible interaction).
Speeds that are closer and closer to the speed of light create more and more interaction with the aether.
What are gravity waves?
Gravity waves are stretching and compressing (shrinking) a part of the painting (after it has been painted) in a certain direction.
What is a Lorentz contraction (Length contraction)?
This is actually a traffic jam (like being stuck in a red traffic light). The first points are slowing and so the points behind them have time to get closer to them.
The whole body (which is comprised from many points) shrinks in the direction of the movement (the more the speed approaches the speed of light).
More thoughts:
Quantum entangled particles are on the same level of “zoom in” – which means they are in the same color palette.
(their movement is slower because they are moving in a strong “zoom”.
It could be that particles can only react with particles which are in the same level of zoom.
That’s why there are particles let’s say Neutrino which are on their own zoom level and so they are not affected by other particles.
This can also explain “quantum tunnelling”, let’s say that the particle passes through a wall, maybe this particle at that moment is in another zoom level than that of the wall’s particles.
The natural movement is the spiral. This is the most common shape in the Mandelbrot set.
When there is a magnetic field this is the natural movement, we see this in pictures from colliding particles in particle accelerators.
https://cds.cern.ch/record/39312
Bubble chamber: colour enhanced tracks
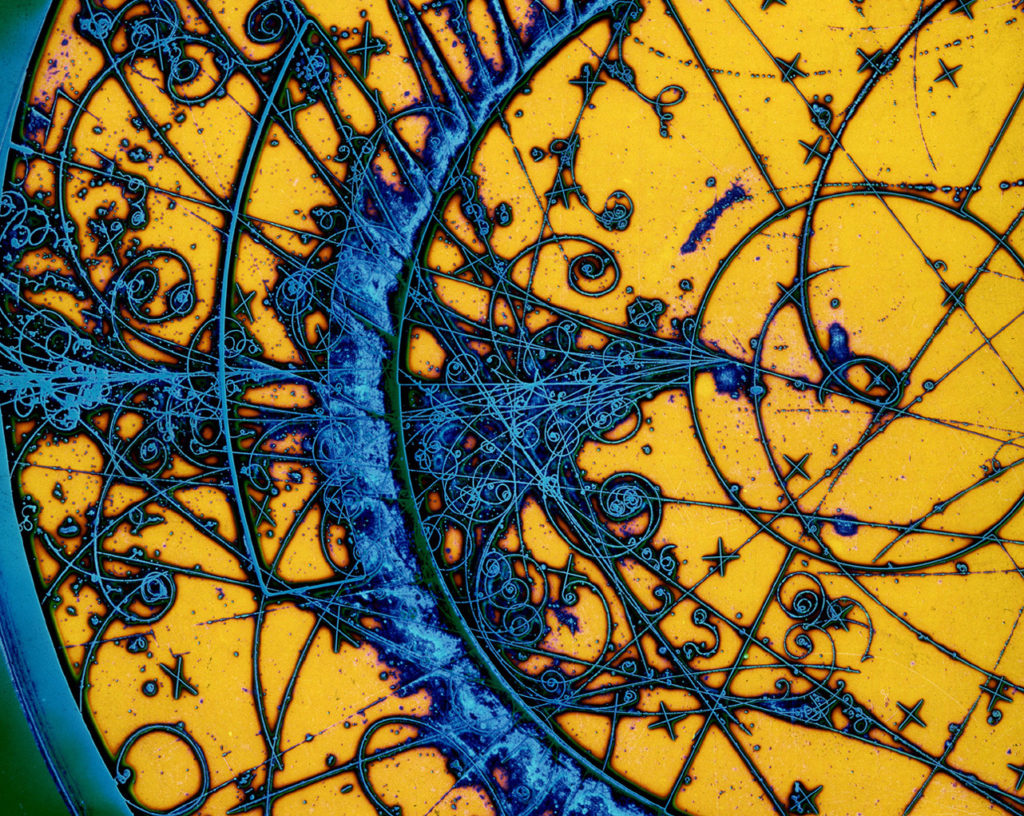
CERN-EX-66954B-1
Focus (NY, USA) (Mar 1994)
The Guardian (UK) : 17 Dec 1993
Original ref.: PI-66954 B
CERN PhotoLab / Experiments and Tracks
CERN-EX-66954B
Thank you very much to CERN for the image, all rights reserved to CERN (C).
A straight line movement happens when two opposite spirals contradict each other.
Usually we have matter which is electrically neutral it has plus and minus (like there is an electron and then next to it a positive “hole”).
The plus pulls to a spiral in one direction, the minus pulls to a spiral in the opposite direction – they cancel each other and a straight line is formed.
But when there is only one kind of charge, then they don’t neutralize each other, and there is a spiral path.
So in these cases we are exposed to the shape of the Mandelbrot set which is under our reality.
Also there are levels of zoom in which there are alternating stripes of black and color.
So maybe this is what makes the turbulence, like “speed bumps” on the road.
Maybe the particle jumps into a deeper level of zoom in this way (and so slows down because of the need for further calculations).
Part 3 – How my theory fits with Quantum Mechanics
In this part I read the book “Wholeness and the Implicate Order” by David Bohm,
https://en.wikipedia.org/wiki/Wholeness_and_the_Implicate_Order
and basically each time he talks about RANDOM put the word CHAOTIC or FRACTAL.
This book was first published in 1980 so it’s 2 years before the famous book by Benoît Mandelbrot
The Fractal Geometry of Nature
https://en.wikipedia.org/wiki/The_Fractal_Geometry_of_Nature
But I was amazed from the amount of places in Bohm’s book that practically saying FRACTAL without saying the word (because the word was not known to Bohm or anybody else for that matter).
So here I collected all the places where Bohm used ideas that are natural to fractals, and as far as I could connected them with the appropriate fractal.
Page 105 (main idea of his book):
Bohm makes a new theory which works non-locally, and quantum mechanics is just an edge case of Bohm’s theory. This edge case is when the local influences are the most important.
On the bottom of page 109:
It’s possible that the vacuum is built in a space filling way that leaves “holes” and then it doesn’t fit any “canonical transformation”, but it DOES fit Sierpiński triangle
https://en.wikipedia.org/wiki/Sierpi%C5%84ski_triangle
and Menger sponge
https://en.wikipedia.org/wiki/Menger_sponge
Page 111-112:
Bohm is trying to turn the uncountable to countable
https://en.wikipedia.org/wiki/Countable_set
by statistical averages.
In my opinion the simplification will be by finding the “strange attractor” (the heart of the fractal),
https://en.wikipedia.org/wiki/Attractor#Strange_attractor
or another option is the few fractals that connect and create the tiny fluctuations (that we can’t calculate completely).
Page 113:
Heisenberg’s uncertainty principle
To us the electron (or any particle) seems to be in one place, but the particle is building objects like we build the Sierpiński triangle through the chaos game:
https://en.wikipedia.org/wiki/Sierpi%C5%84ski_triangle#Chaos_game
Chaos Game – Numberphile
Numberphile
https://www.youtube.com/watch?v=kbKtFN71Lfs
The particle is jumping far from place to place, but we simply see the “concentrated” points (when we look on a large scale).
Page 114:
In Sierpiński triangle there is also an analogy to the uncertainty principle:
The color of the point tells us if the point is on the fractal (white) or not (black).
To know with more and more certainty whether a pixel is black or white we wait more and more time.
BUT
The longer we wait, the less we can track where the “particle” (the current pixel) is right now, because everything is already “scribbled on” (doodled).
There is something similar in Mandelbrot set:
The more and more we would like to know accurate color of a single point,
The less and less we will succeed at the same time to calculate what the whole picture looks like.
Also there is an older idea of mine of a trade-off in the product (the result of multiplication)
“screen resolution” TIMES “color depth” EQUAL “limited graphic memory”.
You can see it in my website for free:
Fractal “Matrix” and Computational Uncertainty Principle
http://www.strulovitz.org/fractal-uncertainty.html
And you can also read this in one of my previous books:
Destiny In Time
https://www.amazon.com/Destiny-Time-Nir-Strulovitz-ebook/dp/B07YNWNB36/
I think that copy-pasting it to here will be disrespectful to you the reader.
Page 114 bottom:
“in all levels of size”
Something that behaves the same on all scales, this sounds very fractal.
A page later Bohm says this doesn’t make sense, but that’s because Bohm didn’t get to know fractals.
Page 123 (I’m quoting and highlighting):
Let us suppose that the [infinity of non-linearly coupled field variables ] is in reality so organized that in each region of space and time associated with any given level of size there is taking place a periodic inner process…
…This periodic process would determine a kind of inner time for each region of space, and it would therefore effectively constitute a kind of local ‘clock’.
Page 126 (I’m quoting again):
Each level will be to some extent be influenced directly by all the other levels, in a way that cannot be fully expressed in terms of their effects on the lower level quantities alone.
Page 131:
SPACE FILLING
Like Menger sponge which is a fractal.
Also in “The Teaching Company” lectures about quantum, she explains that there are places where the particle isn’t passing at all, as opposed to a classic oscillator (like a swing in a playground) that passes through the middle each time it goes from side to side. In the quantum world it skips the middle.
So this again is similar to “the chaos game” where you jump to the next point.
Page 154:
Bohm says some mathematicians especially Wiener thought that Brownian motion is the basic description of nature, and all the movements that look straight are approximations to the real path which is like Brownian motion (I guess like the river mainly flows forward in a more or less straight line despite the movements in all direction in the turbulence inside the water).
I think if one is willing to accept the above explanation, the fractal explanation (I mean that fractals are the basic description of nature) is a much more convincing explanation, and a more useful explanation – fractals are not random noise, they are chaos and they have “laws”.
Page 157:
Bohm says that we cannot analyze the movement in the “world tube” in terms of finer particles, because they are also made of such tubes and so on and so on ad infinitum. Again reminds very much of the self-similarity of fractals.
Page 183:
Hologram!
Each part of the picture contains the essence of the entire complete picture (but in less details).
This is exactly like things are with fractal because of the self-similarity (in a fractal is not with less details but with a little “twist”).
Page 188:
Rotating a drop inside a viscous transparent liquid. This is called Taylor–Couette flow:
https://en.wikipedia.org/wiki/Taylor%E2%80%93Couette_flow
check out this very cool video:
Unmixing Color Machine (Ultra Laminar Reversible Flow) – Smarter Every Day 217
https://www.youtube.com/watch?v=j2_dJY_mIys
SmarterEveryDay
So this is just like:
Stephen Smale Horseshoe map
Which is a fractal!
https://en.wikipedia.org/wiki/Horseshoe_map
which is like folding dough in half and then kneading it, and then repeating the process over and over again.
So if you track a point on the dough its location is a fractal.
Page 192:
“then we will see what appears to be ‘solid’ object…because the eye is not sensitive…”
This is said about a quick rotation of the rotating cylinder in the Taylor–Couette system with a few droplets that look like animation.
But notice how the same description fits for example the Menger sponge fractal which seems solid to us because our discernment ability is limited (finite).
Page 194:
Bohm talks about painting a whole picture in the technique that we just talked about (rotating the cylinder fast).
This is like painting the Sierpiński triangle using just one pixel which is lit in any given time, by relying on the human “persistence of vision”.
This is like the John Wheeler’s “One-electron universe”, which I will explain soon.
Page 199:
The mathematics doesn’t only describe physics but also creates physics.
This is like a fractal is created by a mathematical formula or equation.
Page 202:
For some changes Bohm uses the term “metamorphosis”.
“This word indicates that the change is much more radical than the change of position of orientation of a rigid body, and that it is in certain ways more like the change from caterpillar to buttefly (in which everything alters in a thorough going manner while some subtle and highly implicit features remain invariant)…”
This is like the changes you will see in the following fracal “morpher” videos of the demoscene, and in the video where Numberphile show that the Mandelbrot set contains inside it all the possible Julia sets. The change from one Julia set to another is what Bohm calls “metamorphosis”.
Page 211:
“…the whole of space can then be treated through the use of a very large number of overlapping coordinate ‘patches’.”
…
“‘the law of the whole’ is such that similar structures cab be built on each order.”
Doesn’t this sound like different orders of magnitude of a fractal?
Okay so by now I hope you have a feeling that reality is built from fractals. But what does it look like? So instead of a lengthy verbal description I wish to explain through videos, especially the demos and intros I watched as a teenager.
https://en.wikipedia.org/wiki/Demoscene
Demos are the ultimate art form of “real time”* programming, short presentations that combine graphical effects and electronic music. The special thing is that everything you see and hear is calculated by the computer on the fly and not like in a movie where every frame is calculated for hours on lots of expensive work stations. In demos the challenge is what a normal computer is able to do as you watch and listen. Demos always try to push the envelope of the computer can do, like hackers but it’s all about original art. The Demoscene are the parties which are fun competitions where groups of these programmers and artists meet and show off their skills to each other and to their fans.
To force this kind of programming, so that people will not “hide” a video inside the program, some categories of demos are very small. For example “intro” on our table (intro is a small sized demo) is in the 64 Kilobyte category, so all the things you see and hear for a few minutes are squeezed down to the size of one small 320×200 picture! The second one on our table is in the 1 Kilobyte, so what you see there is squeezed into the size of a 200 words text! There are even demo category for 256 bytes. This is so small it’s the size needed to store 64 characters. Less than half of a Twitter message!
So since now these masterpieces are on Youtube in video form, you can watch them if you have the link, and this is what we will do here.
PLEASE DON’T JUST WATCH THE FROZEN PICTURE –
YOU HAVE TO SEE THEM MOVE AND CHANGE TO UNDERSTAND WHAT I MEAN!
(ESPECIALLY EVERY TIME IT SAYS “MORPHER”)
Physics effect Demos effect (fractal)
EPR paradox
Non casual correlations
Influence from afar
Quantum entanglement Drift by Wildlight
https://www.youtube.com/watch?v=4lB55Q92vB8
Minute 1:09 to 1:46
Fractal morpher
Discrete packets
Indivisibility of the quantum action Untraceable by TBC
https://www.youtube.com/watch?v=qQNIKOD6WnY
Minute 1:26 to 1:54
There are separate “corridors” inside the Menger sponge
Menger sponge has ZERO mass but infinite surface area, so it’s a very good candidate for our fractal aether.
Wave-particle duality
The wave is periodic in time.
In the places where the wave is “concentrated” (where there is a lot of matter) it looks to us from far away like a particle. Crystal Dream 2 by Triton
https://www.youtube.com/watch?v=3jyTysTORNQ
minute 3:15 to 3:41
fractal zoom
OK here is an ALTERNATIVE theory where our progress is not ACROSS the fractal but INTO the fractal. I’m also leaving it here, because maybe it will give insight to somebody who can improve it.
a fractal is periodic in scale.
Where there are points that are on the fractal (there are lots of details if we zoom) this is called matter.
Other places (in which we can zoom as much as we want but it will remain in the same color) are empty.
The “path” of our camera is going through time segments that have lots of details, if they are close to each other in the sense of time (quickly one after the other) then this looks to us like particles.
WE could choose a camera path in which we would meet another small Mandelbrot set first, so the meetings would be frequent so this would be a particle.
An influence between the viewer and the observed on small scale.
In bohm’s terminology this is “properties of matter as statistical potentialities”.
Verses by Electromotive Force
https://www.youtube.com/watch?v=JT4MsEleaZc
Minute 0:34 to 0:55
IFS morpher
By the way in minute 3:21 to 3:31 there is a Julia morpher like in Drift.
Another example of the same thing:
Live evil by Mandula
https://www.youtube.com/watch?v=wSAkUZvhyv4
Minute 3:26 to 4:00
The fractal flowers
Speed of light as ultimate barrier
That’s the ALTERNATIVE theory again, where our progress is not ACROSS the fractal but INTO the fractal.
Movement from one place to the other in the fractal means zoom out to the common zoom level for the two locations (the origin and the destination), and then move across at that same level, and then do zoom in. Stoned by Dust
https://www.youtube.com/watch?v=oayvAoENh9c
Minute 0:06 to 0:40
This is a “drugged” Mandelbrot zoom so:
It goes in to one place and then comes out
It goes in to another place then comes out
OK here I’m continuing our last line at the table, because if I’ll write this inside a narrow column of a table it will take a whole page of the book so here goes:
Remember this is in the ALTERNATIVE theory, not my main theory, but it’s still interesting.
Zoom in = time flows forward = ordinary matter.
Zoom out = time flows backward = anti-matter.
(Maybe there is a connection to Bohm’s unfolding = zoom in, and to Bohm’s folding = zoom out).
Okay so think about the “speed of light” as the speed of zooming in.
It takes more time to calculate the zoom in than it takes to reconstruct the zoom out (which is calculation that has already been done).
This is why there is more ordinary matter in the universe than there is anti-matter in the universe!
So it could be that John Wheeler was right:
“Feynman, I know why all electrons have the same charge and the same mass” “Why?” “Because, they are all the same electron!”
https://en.wikipedia.org/wiki/One-electron_universe
In his Noble winning speech, Richard Feynman confessed that he “stole” the idea that positrons (anti-particle of electron) are just electrons moving backwards in time.
So in my opinion the reason why we have a lot of electrons (ordinary matter) and few positrons (anti-matter) is because the particle exists in our universe much less time as an anti-particle, because it’s quicker to calculate the zoom out.
Okay now back to my regular theory (we’ve finished with my ALTERNATIVE theory for now).
Okay so now we’re going to the heart of my theory, so you must see the following video on Youtube, it’s amazing, the part I ask you to see is from minute 15:18 to minute 15:44
https://www.youtube.com/watch?v=FFftmWSzgmk
What’s so special about the Mandelbrot Set? – Numberphile
Numberphile
Please please see the video moving and changing, this is just a “frozen” image:
Notice where his mouse is on the right side of the screen, near the “cleavage” of the big shape.
Notice on the top left the matching Julia set.
So what Ben Sparks basically does in that part of the video, he turns the software into “Julia set mode”, and so the computer shows us a small preview of what the small Julia fractal (that matches that specific point on the big Mandelbrot fractal, the point where the user mouse is right now) looks like.
The Mandelbrot set is like a map of all the possible Julia sets.
Another video on Youtube in the same subject that you must see is this video which is also amazing, here you need what she explains on minute 4:45
Filled Julia Set
https://www.youtube.com/watch?v=oCkQ7WK7vuY
Numberphile2
Featuring Dr Holly Krieger from MIT.
OK so what the amazing Dr. Holly Krieger is saying, is that you pick a point that seems to be on the Mandelbrot set, and you check what kind of Julia set you receive from that point: the connected kind like the “Douady rabbit” that you see in the “bubble” on the top right…?
https://en.wikipedia.org/wiki/Douady_rabbit
OR the disconnected (“scattered”) kind like the groups of dots that you see in the “bubble” below it?
The points that produce connected Julia shape are IN the Mandelbrot set, but the points that produce a disconnected Julia shape (that look like dots) are NOT in the Mandelbrot set.
This is another way to define the Mandelbrot set.
This is very relevant to us, because next to each other there will be points that have connectedness which means connection between far away points which means quantum entanglement;
And there will be points in which each point is operating on her own which means there is NO quantum entanglement.
